What's "Integer," Precious?

In short, an integer is just a number. It must be a whole number (not a fraction, or decimal) and integers can be negative.

Examples of POSITIVE integers: 1, 2, 3, 40, 100, 3,429, 62, 7, 1,000,000

Examples of NEGATIVE integers: -1, -4, -67, -100, -1000, -5,673, -12

Let's do an exercise now. We'll see if you can locate integers, and weed out the ones that aren't integers at all!
EXERCISE 1: Is This An Integer??

1. 1

2. 66

3. 5½

4. -7

5. 7

6. 7.7

7. one thousand

8. -2¼

9. 0

10. negative one

Your answers, M'ladies and M'lords:

1. Yes (1 is a whole number)

2. Yes (66 is a whole number)

3. No (Half of something means that you need the other half to complete it, it being that whole number!)

4. Yes (-7 is negative, but is also whole)

5. Yes (7 is a whole number)

6. No (7.7 has a decimal, meaning the number is incomplete and not whole)

7. Yes (I wrote it out in words, not a number, but that doesn't change 1000 from being whole)

8. No (A fourth is a fraction, meaning it's not a whole number)

9. Yes (0 can be confusing because zero is neither negative nor positive, but it is whole

10. Yes (If you write negative one in number, -1, you can easily see it is a whole number)

factmonster.com has given us an interesting set of facts and explanations regarding integers.

That's all the in(teger)formation you get today! If you want to see more about integers, have a good definition, or found a funny integer pic you want to share here on the blog, shoot me an email at: artfromnike@gmail.com

Squared and Cubed

This is a simple post! Hooray!

Don't be frightened by the term "squared" or cubed," because they're only names for exponents*
*Read the Exponents post first!!

7 to the second power ; 7➁ = squared

7 to the third power ; 7➂ = cubed

So the exponent 2 means "squared" and the exponent 3 means "cubed". Easy as pi...er, pie!

Want to do those problems for practice? Of course you don't, but here they are:

7➁ means 7 x 7 (seven, two times) which = 49

7➂ means 7 x 7 x 7 (seven, three times) which = 343

Now you are free to stop reading this stupid math blog and hate me for making it ☺

Exponents (Powers): A Simple Explanation

Remember mates, I can't figure out how to use exponents on a computer yet, so these will stand in for exponents: ➀ ➁ ➂ ➃ ➄ ➅ ➆ ➇ ➈ ➉ (Sorry for the inconvenience!)

Here's what the numbers in a problem with exponents, or "powers" are called.

I have seen some classmates misuse the exponent. This problem seems to be very common. What happens is: Students take the exponent and multiply it with the base number. So going by this picture, they would come up with -

8 (base number) x ➁ (exponent) = 16

BUT THIS ISN'T CORRECT!

Let's have a brief interview with this exponent ➁ from the problem.

Nike: Hey, exponent, how are you today?

Exponent: Feeling powerful Nike, thanks for asking.

N: Good! Now exponent, can you remind all the viewers what made you so famous?

E: Well I help Base Numbers multiply themselves.

N: How do you do this, exactly?

E: It's simple, really: see that 8 there?

N: Yes.

E: Well I'm not telling number 8 to "times" itself by me.

N: Oh?

E: A number has to rely on itself. I'm helping the 8 to become a bigger number. I'm saying, "8, times yourself BY yourself!"

N: So in other words...

E: I'm only telling the 8 to times ITSELF twice. 8 x 8 = 64.

N: So what if a ➆ were involved instead of you, ➁?

E: Well I don't much care for ➆, becuase ➆ ate ➈.

N: Oh Exponent, you crack me up!

E: Let's try a more understandable problem. Let's invite Exponent-Babe to show us how to do 2➂.

N: That's a great idea: Exponent-Babe is smart and sexy!

So there we have it. Between the talking exponent and Exponent-Babe's examples, you should have a better understanding of how they work. Here are two easy and quick tips to remember:

☼ DON'T multiply the base number with the exponent!
☼ Multiply the base number by itself; the exponent just tells you how many times to multiply it by.

It's dangerous to go alone; take this chart! (save it to your computer, it will help you!)

Questions, comments, concerns, or funny pictures of cats: artfromnike@gmail.com

Equation vs Expression

Two 3-syllable E words that end in "ion" ??! NOOOOO- oh wait, I forgot, there's a simple trick to remembering the two!

An EQUATION always has an EQUAL SIGN like this

2 + 2 = 4

An EXPRESSION expresses the problem without the equal symbol found in an equation

2 + x

Which problems are equations, and which are expressions?

A. 1x - 2

B. 4 - 2 = 2

C. 4 - (2)

D. -1 - (-3) = x

E. 5 + n = p

A. Expression

B. Equation

C. Expression

D. Equation

E. Equation

B, D and E are all "Equations" because of the equal sign. Remember: "equation," "equal."

Sum - Difference - Product - Quotient

In early math, like Pre-Algebra, it's our job to understand the most basic functions of adding, subtracting, multiplying and dividing. Take a look at this short, informative, and incredibly hip video from the Youtube!

What the video says is simple, repetitive,repetitive and not only repetitive, but repetitive. Fortunately, repetition is what you need when you're doing basic algebra. You're going to need to know how to add, subtract, multiply and divide when college takes you to higher level math.

If you didn't watch the video because you don't want to be hip (it's okay, math needs squares) here's what the video was telling you:

ADD numbers and the answer is the SUM

2 + 2 = 4

SUBTRACT numbers and the answer is the DIFFERENCE

6 - 3 = 3

MULTIPLY numbers and the answer is the PRODUCT

1 x 9 = 9

DIVIDE numbers and the answer is the QUOTIENT

8 ÷ 2 = 4

PEMDAS (Order of Operations)

(PEMDAS) is an acronym used to help students in their exponentially difficult journeys through Multiplying, Diving, Adding and Subtracting their way through general education.

P stands for Parentheses

E stands for Exponents

M stands for Multiplication

D stands for Division

A stands for Addition

S stands for Subtraction

This process is called the Order of Operations. Let's delve right into a problem from the book, Chapter 1.7 Problem 49:

32 ÷ 4 - 3

♥ First, we check to see if there are parentheses. There are none, so we skip this step.
♥ Next, are there exponents? No. Skip this step as well.
♥ Multiplication? No.
♥ Division? Eureka! There is division here so we have to do that first.

32 ÷ 4 = 8

♥ Here is an invisible (but equally as important) step: rewrite the problem with your new solution to the division portion.

8 - 3

♥ Is there any addition to be done? No. Skip this step.
♥ Lastly, is there subtraction? Yes! Let's subtract!

8 - 3 = 5

♥ There is nothing more to be done, and you're left with a 5.

The Answer Is 5

Now we're going to try a harder one that uses the entirety of PEMDAS.
**NOTE that I can't get exponents to work, so exponents will be represented in a circle like this: ➀ (Sorry!)
This problem is from the class handout "Section 1.7 Part II - Order of Operations: Problem 7).

35 ÷ [3➁ + (9 - 7) - 2➁] + 10 x 3

Yowza! It looks scary! But just as this problem is your beast to be slain, PEMDAS is your Excalibur.
♥ First, we do parentheses. See the brackets? Everything in there is going to be done first. Brackets are like parentheses and essentially work the same way, and usually contain actual parentheses within them. Let's solve the parentheses inside the brackets.

(9 - 7 = 2)

35 ÷ [3➁ + 2 - 2➁] + 10 x 3

♥ Exponents are next, and they're all inside the brackets.
♥ ** GO LEFT TO RIGHT when there are two of the same type of thing to do, like these exponents.

3➁ = 9

2➁ = 4

35 ÷ [9 + 2 - 4] + 10 x 3

♥ Now continue to solve inside of the brackets, as they serve as parentheses.
♥ We've done parentheses and exponents, and there are no multiplication or division problems in the brackets, so we do addition.

9 + 2 = 11

35 ÷ [11 - 4] + 10 x 3

♥ Finally, finish off the brackets with subtraction.

11 - 4 = 7

35 ÷ [7] + 10 x 3

♥ Now that there is no work to be done within parentheses (or brackets!) we move PEMDAS onto the rest of the problem. No more parentheses, no exponents, but there is multiplication.

10 x 3 = 30

35 ÷ [7] + 30

♥ After multiplication comes division. Let's divide 7 into 35.

35 ÷ 7 = 5

5 + 30

♥ In this case, our only, and final, step, is addition. Let's solve this bad boy.

5 + 30 = 35

ANSWER = 35

Awesome! Now, 2 last pieces of advice. Firstly, always go left to right. Like, if you have an order of operations problem with two sets of exponents, solve the left one first. And my other advice is, use a mnemonic device to help you remember the order of PEMDAS. I'll leave you with some you can choose to use choose to lose!

♥ Please Excuse My Dear Aunt Sally (classic!)
♥ Print Evan's Microsoft Document And Send
♥ Princess Emily's Mom Died...Aww, Sad ☹

Let's Get Started!

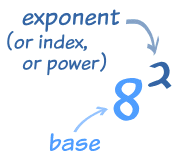
Welcome to Mathcabulary!!

What is Mathcabulary? It is a series of words and terms frequented in MATH 217 (or Pre-Algebra). Let's talk about what you're going to expect here.

As solemnly sworn, I created a vocabulary mosh-pit for basic math students at Butte Community College (or students anywhere!) Accessing Mathcabulary will give you access to words like "integer" or "product," words that will end up on quizzes, tests and homework. These are words all students should understand before charging into higher math.

This is me, Nike.


I created Mathcabulary because of a very bad experience. One summer, I took this very class while I was seeing multiple doctors who were all oblivious and ignorant toward my medical condition, which is autoimmune. One particular doctor took me off of my anxiety medication and replaced it with an antidepressant, even though I don't have clinical (or non-clinical)depression. While taking said pills, I forgot all of the things I learned in MATH 217, and even permanently lost a portion of my life, unable to remember anything, all because of a 10 minute visit to a bad doctor. Now, I take doctors and medication very seriously, and I take my studies seriously as well. Mathcabulary will ensure that students have somewhere to go when they forget what "greater than" means. This is a tool that would have helped me, and I know it will help others.

FINDING WORDS AND TERMS

It's okay if you're looking for a word and you aren't computer-savvy. Here's the magic code: Ctrl+F. If you press "control" and "F" at the same time, a search bar will pop up in the upper right hand corner of the page. Type in a specific word, like "substitution" and the page will take you to the word you typed which will be highlighted. You can also use this tool to search for a general topic; if you want to know random, helpful things about multiplication, Ctrl+F "multiplication" and the page might take you to several things involving multiplying. **If you can't find the word you're searching for, check to see if the word is located in a different month's vocab: on the blog's upper right hand corner under "About Me," there is a BLOG ARCHIVE where you can click and scroll down to different months, which will have different words.

This should do the trick. But if the term you want isn't on my blog and you'd like an explanation, email me and I'll put it up ASAP!

ALT Codes

An ALT code is a code that allows you to use math symbols not found on a standard keyboard. For example, check out this awesome division sign ÷ or how I can type ¼ instead of a giant 1/4.

Here is the link: http://usefulshortcuts.com/alt-codes/instructions-for-using-alt-Codes.php

But remember, I don't condone doing homework via Microsoft Word or Open Office as fun as using these codes may be. I just want to share this tool. Homework is a paper thing, so if you print out your homework and turn it into Tamsen (or whomever your teacher is) and say "Nike taught me this!" I will deny it and link your teacher to this testimony. Booyah.

Get More Help With Basic Math

Workshops are held all the time in the CAS! These are 50 minute sessions where you go into a room and learn about math you've frequently studied. This is extraordinarily helpful for MATH 217 students, because it's like doing a review of everything you just covered in class. The tutor will answer all of your questions regarding math, and you will practice on worksheets, on the board, alone and with others.

Need help finding this awesome tutoring shizznit? First, ask your teacher for a leaflet with the times the sessions happen. Find a time that works for you. Directions? Go to the Learning Resource Center (LRC) located across from the science building, and sandwiched in between the gardening/welding section and the MC/library part of campus. Go up to the second floor. To the right is the CAS, or Center for Academic Success. Ask about their tutoring sessions for MATH 217. The person at the desk will log your student ID number into the computer, so have that ready. Then they will direct you to the room where you'll be.

Please, make sure to sign up for CAS as a class. This is SUPER DUPER IMPORTANT okay? You sign up online like you did for your other classes; you add the class to your list of other classes, and yes it's FREE. It will look like this: EDUC 310 - Supervised Tutoring (M3287). Signing up is important because it logs you, as a student, into the system, and if students keep signing up for the free and awesome tutoring CAS provides, The Man will keep paying for it, and we can keep CAS open.

CONTACT ME BABY!

Remember, anyone who wants to be a part of this blog to help absolutely can! If you have a better definition, some links, cute math pictures, great examples, adorable math jokes, or anything helpful you want to share, please email me and I'll do my best to put your stuff up. Also, if a problem is wrong, or you think something could be better, tell me and I'll check it out and fix it, if it needs fixing.

Questions, comments, concerns and creativity go to: artfromnike@gmail.com

After suming it all up, again I say welcome to Mathcabulary. It will be exponentially fun.